Kurt Gödel Offers Proof of the Existence of God
The first version of the ontological proof in Gödel’s papers is dated “around 1941”. Gödel is not known to have told anyone about his work on the proof until 1970, when he thought he was dying. In February, he allowed Dana Scott to copy out a version of the proof, which circulated privately. In August 1970, Gödel told Oskar Morgenstern that he was “satisfied” with the proof, but Morgenstern recorded in his diary entry for 29 August 1970, that Gödel would not publish because he was afraid that others might think “that he actually believes in God, whereas he is only engaged in a logical investigation (that is, in showing that such a proof with classical assumptions (completeness, etc.) correspondingly axiomatized, is possible).”[2]Gödel died January 14, 1978. Another version, slightly different from Scott’s, was found in his papers. It was finally published, together with Scott’s version, in 1987.[3]
Morgenstern’s diary is an important and usually reliable source for Gödel’s later years, but the implication of the August 1970 diary entry—that Gödel did not believe in God—is not consistent with the other evidence. In letters to his mother, who was not a churchgoer and had raised Kurt and his brother as freethinkers,[4] Gödel argued at length for a belief in an afterlife.[5] He did the same in an interview with a skeptical Hao Wang, who said: “I expressed my doubts as G spoke […] Gödel smiled as he replied to my questions, obviously aware that his answers were not convincing me.”[6] Wang reports that Gödel’s wife, Adele, two days after Gödel’s death, told Wang that “Gödel, although he did not go to church, was religious and read the Bible in bed every Sunday morning.”[7] In an unmailed answer to a questionnaire, Gödel described his religion as “baptized Lutheran (but not member of any religious congregation). My belief is theistic, not pantheistic, following Leibnizrather than Spinoza.”[8]
The proof uses modal logic, which distinguishes between necessary truths and contingent truths. In the most common semantics for modal logic, many “possible worlds” are considered. A truth is necessary if it is true in all possible worlds. By contrast, a truth is contingent if it just happens to be the case, for instance, “more than half of the planet is covered by water”. If a statement happens to be true in our world, but is false in another world, then it is a contingent truth. A statement that is true in some world (not necessarily our own) is called a possible truth.
From axioms 1 through 4, Gödel argued that in some possible world there exists God. He used a sort of modal plenitude principle to argue this from the logical consistency of Godlikeness. Note that this property is itself positive, since it is the conjunction of the (infinitely many) positive properties.
Then, Gödel defined essences: if x is an object in some world, then the property P is said to be an essence of x if P(x) is true in that world and if P entails all other properties that x has in that world. We also say that x necessarily exists if for every essence P the following is true: in every possible world, there is an element y with P(y).
Since necessary existence is positive, it must follow from Godlikeness. Moreover, Godlikeness is an essence of God, since it entails all positive properties, and any nonpositive property is the negation of some positive property, so God cannot have any nonpositive properties. Since any Godlike object is necessarily existent, it follows that any Godlike object in one world is a Godlike object in all worlds, by the definition of necessary existence. Given the existence of a Godlike object in one world, proven above, we may conclude that there is a Godlike object in every possible world, as required.
From these hypotheses, it is also possible to prove that there is only one God in each world by Leibniz’s law, the identity of indiscernibles: two or more objects are identical (are one and the same) if they have all their properties in common, and so, there would only be one object in each world that possesses property G. Gödel did not attempt to do so however, as he purposely limited his proof to the issue of existence, rather than uniqueness. This was more to preserve the logical precision of the argument than due to a penchant for polytheism. This uniqueness proof will only work if one supposes that the positiveness of a property is independent of the object to which it is applied, a claim which some have considered to be suspect[who?].
To formalize the argument sketched above, the following definitions and axioms are needed:
-
Definition 1: x is God-like if and only if x has as essential properties those and only those properties which are positive
-
Definition 2: A is an essence of x if and only if for every property B, x has B necessarily if and only if A entails B
-
Definition 3: x necessarily exists if and only if every essence of x is necessarily exemplified
-
Axiom 1: Any property entailed by—i.e., strictly implied by—a positive property is positive
-
Axiom 2: A property is positive if and only if its negation is not positive
-
Axiom 3: The property of being God-like is positive
-
Axiom 4: If a property is positive, then it is necessarily positive
-
Axiom 5: Necessary existence is a positive property
Axiom 1 assumes that it is possible to single out positive properties from among all properties. Gödel comments that “Positive means positive in the moral aesthetic sense (independently of the accidental structure of the world)… It may also mean pure attribution as opposed to privation (or containing privation).” (Gödel 1995). Axioms 2, 3 and 4 can be summarized by saying that positive properties form a principal ultrafilter.
From these axioms and definitions and a few other axioms from modal logic, the following theorems can be proved:
-
Theorem 1: If a property is positive, then it is consistent, i.e., possibly exemplified.
-
Theorem 2: The property of being God-like is consistent.
-
Theorem 3: If something is God-like, then the property of being God-like is an essence of that thing.
-
Theorem 4: Necessarily, the property of being God-like is exemplified.
Symbolically:
![\begin{array}{rl}\text{Ax. 1.} & \left\{P(\varphi) \wedge \Box \; \forall x[\varphi(x) \to \psi(x)]\right\} \to P(\psi) \\\text{Ax. 2.} & P(\neg \varphi) \leftrightarrow \neg P(\varphi) \\\text{Th. 1.} & P(\varphi) \to \Diamond \; \exists x[\varphi(x)] \\\text{Df. 1.} & G(x) \iff \forall \varphi [P(\varphi) \to \varphi(x)] \\\text{Ax. 3.} & P(G) \\\text{Th. 2.} & \Diamond \; \exists x \; G(x) \\\text{Df. 2.} & \varphi \text{ ess } x \iff \varphi(x) \wedge \forall \psi \left\{\psi(x) \to \Box \; \forall y[\varphi(y) \to \psi(y)]\right\} \\\text{Ax. 4.} & P(\varphi) \to \Box \; P(\varphi) \\\text{Th. 3.} & G(x) \to G \text{ ess } x \\ \text{Df. 3.} & E(x) \iff \forall \varphi[\varphi \text{ ess } x \to \Box \; \exists y \; \varphi(y)] \\ \text{Ax. 5.} & P(E) \\ \text{Th. 4.} & \Box \; \exists x \; G(x) \end{array}](https://i0.wp.com/upload.wikimedia.org/math/a/b/4/ab47f643c7918fda58089c7f5129a183.png)
There is an ongoing open-source effort to formalize Gödel’s proof using various theorem provers and proof assistants. The formalized proof of God’s existence made headlines in German newspapers.
__________________________________________________________

ABC NEWS
Computer Scientists ‘Prove’ God Exists
Oct. 27, 2013
By David Knight, SPIEGEL
Two scientists have formalized a theorem regarding the existence of God penned by mathematician Kurt Gödel. But the God angle is somewhat of a red herring — the real step forward is the example it sets of how computers can make scientific progress simpler.
As headlines go, it’s certainly an eye-catching one. “Scientists Prove Existence of God,” German daily Die Welt wrote last week.
But unsurprisingly, there is a rather significant caveat to that claim. In fact, what the researchers in question say they have actually proven is a theorem put forward by renowned Austrian mathematician Kurt Gödel — and the real news isn’t about a Supreme Being, but rather what can now be achieved in scientific fields using superior technology.
When Gödel died in 1978, he left behind a tantalizing theory based on principles of modal logic — that a higher being must exist. The details of the mathematics involved in Gödel’s ontological proof are complicated, but in essence the Austrian was arguing that, by definition, God is that for which no greater can be conceived. And while God exists in the understanding of the concept, we could conceive of him as greater if he existed in reality. Therefore, he must exist.
Even at the time, the argument was not exactly a new one. For centuries, many have tried to use this kind of abstract reasoning to prove the possibility or necessity of the existence of God. But the mathematical model composed by Gödel proposed a proof of the idea. Its theorems and axioms — assumptions which cannot be proven — can be expressed as mathematical equations. And that means they can be proven.
Proving God’s Existence with a MacBook
That is where Christoph Benzmüller of Berlin’s Free University and his colleague, Bruno Woltzenlogel Paleo of the Technical University in Vienna, come in. Using an ordinary MacBook computer, they have shown that Gödel’s proof was correct — at least on a mathematical level — by way of higher modal logic. Their initial submission on the arXiv.org research article server is called “Formalization, Mechanization and Automation of Gödel’s Proof of God’s Existence.”
The fact that formalizing such complicated theorems can be left to computers opens up all kinds of possibilities, Benzmüller told SPIEGEL ONLINE. “It’s totally amazing that from this argument led by Gödel, all this stuff can be proven automatically in a few seconds or even less on a standard notebook,” he said.
The name Gödel may not mean much to some, but among scientists he enjoys a reputation similar to the likes of Albert Einstein — who was a close friend. Born in 1906 in what was then Austria-Hungary and is now the Czech city of Brno, Gödel later studied in Vienna before moving to the United States after World War II broke out to work at Princeton, where Einstein was also based. The first version of this ontological proof is from notes dated around 1941, but it was not until the early 1970s, when Gödel feared that he might die, that it first became public.
Now Benzmüller hopes that using such a headline-friendly example can help draw attention to the method. “I didn’t know it would create such a huge public interest but (Gödel’s ontological proof) was definitely a better example than something inaccessible in mathematics or artificial intelligence,” the scientist added. “It’s a very small, crisp thing, because we are just dealing with six axioms in a little theorem. … There might be other things that use similar logic. Can we develop computer systems to check each single step and make sure they are now right?”
‘An Ambitious Expressive Logic’
The scientists, who have been working together since the beginning of the year, believe their work could have many practical applications in areas such as artificial intelligence and the verification of software and hardware.
Benzmüller also pointed out that there are many scientists working on similar subject areas. He himself was inspired to tackle the topic by a book entitled “Types, Tableaus and Gödel’s God,” by Melvin Fitting.
The use of computers to reduce the burden on mathematicians is not new, even if it is not welcomed by all in the field. American mathematician Doron Zeilberger has been listing the name Shalosh B. Ekhad on his scientific papers since the 1980s. According to the New York-based Simons Foundation, the name is actually a pseudonym for the computers he uses to help prove theorems in seconds that previously required page after page of mathematical reasoning. Zeilberger says he gave the computer a human-sounding name “to make a statement that computers should get credit where credit is due.” “human-centric bigotry” on the part of mathematicians, he says, has limited progress.
Ultimately, the formalization of Gödel’s ontological proof is unlikely to win over many atheists, nor is it likely to comfort true believers, who might argue the idea of a higher power is one that defies logic by definition. For mathematicians looking for ways to break new ground, however, the news could represent an answer to their prayers.
link athttp://abcnews.go.com/Technology/computer-scientists-prove-god-exists/story?id=20678984
__________________________________________________________
Leonhard Euler Offers Proof of the Existence of God
Leonhard Euler (April 15,1707 – September 18, 1783) was a pioneering Swiss mathematician and physicist who made important discoveries in fields as diverse as infinitesimal calculus and graph theory. Euler also introduced much of the modern mathematical terminology and notation, particularly for mathematical analysis, such as the notion of a mathematical function, and he is renowned for his work in mechanics, fluid dynamics, optics, and astronomy. He spent most of his adult life in St. Petersburg, Russia and in Berlin, Prussia.
Much of what is known of Euler’s religious beliefs can be deduced from his Letters to a German Princess and an earlier work, Rettung der Göttlichen Offenbahrung Gegen die Einwürfe der Freygeister (Defense of the Divine Revelation against the Objections of the Freethinkers). These works show that Euler was a devout Christian who believed the Bible to be inspired; the Rettung was primarily an argument for the divine inspiration of scripture.
There is a famous legend inspired by Euler’s arguments with secular philosophers over religion, which is set during Euler’s second stint at the St. Petersburg academy. The French philosopher Denis Diderot was visiting Russia on Catherine the Great’s invitation. However, the Empress was alarmed that the philosopher’s arguments for atheism were influencing members of her court, so Euler was asked to confront the Frenchman. Diderot was informed that a learned mathematician had produced a proof of the existence of God; he agreed to view the proof as it was presented in court. Euler appeared, advanced toward Diderot, and in a tone of perfect conviction announced the following non-sequitur: “Sir, \frac{a+b^n}{n}=x, hence God exists—reply!” Diderot, to whom (says the story) all mathematics was gibberish, stood dumbstruck as peals of laughter erupted from the court. Embarrassed, he asked to leave Russia, a request that was graciously granted by the Empress.
link athttp://www.oddee.com/item_98822.aspx
__________________________________________________________
Mathematicians and scientists seemed to ignore a simple equation familiar to all grammar school children: 0 + 0 = 0
For the Big Bang Theory to work, this always-true equation had to be false—at least once—and if this basic equation is unreliable, so is the rest of the math used to prove the Big Bang.
Dr. Adrian Rogers, a pastor and Bible teacher from Memphis, TN, once challenged the Big Bang Theory by putting the 0 + 0 = 0 equation into more specific terms: “How can nobody plus nothing equal everything?”
__________________________________________________________
A Proof Even Better Than Math
I’m no math expert, but for more than 40 years I have studied how people act and why they do what they do. Human nature is remarkably consistent, regardless of the culture or era in history. For me, the best proof of God depends on one cowardly fisherman.
Simon Peter, Jesus’ closest friend, denied knowing Jesus three times in the hours before the crucifixion. If any of us had faced possible crucifixion, we probably would have done the same thing. Peter’s so-called cowardice was completely predictable. It was human nature.
But it was what happened later that causes me to believe. Not only did Peter come out of hiding after Jesus’ death, he began preaching the resurrection of Christ so loudly that the authorities threw him in jail and had him severely beaten. But he got out and preached all the more!
And Peter wasn’t alone. All the apostles who had been cowering behind locked doors spread out across Jerusalem and the surrounding area and began insisting that the Messiah had been raised from the dead. In the following years, all of Jesus’ apostles (except Judas who hanged himself and John, who died of old age) were so fearless in proclaiming the gospel that they were all murdered as martyrs.
That is simply not human nature.
One thing and one thing only can explain it: These men had encountered the real, solid, bodily-resurrected Jesus Christ. Not a hallucination. Not mass hypnosis. Not looking in the wrong tomb or any other silly excuse. The flesh and blood risen Christ.
That’s what my father believed and that’s what I believe. I don’t have to do the math to know that my Savior lives, and because He lives, I fully expect to see both Him and my father again some day.
link athttp://christianity.about.com/od/newchristians/a/proofgodexists.htm
__________________________________________________________
POSITIVE PROOF OF GOD”S EXISTENCE!!!
I have met him, he loves me and he lives within me!

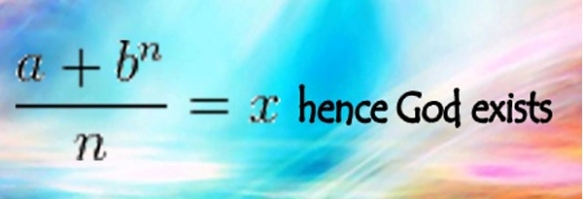
Pingback: Godel’s Argument for Existence of God | Beilsy K Isaac
Attn: Dr. Richter DasMeerungeheuer
Logical proof of Monotheism & the Holographic Universe explained.
Theos – The Supreme Good
Time must exist before matter can be created and only an animate entity can conceive of space-time; time must be a stabilized and uniform condition before matter can form thus, monotheism is a Truth.
‘The Universal Laws of Physics’ and the characteristics of elements which are both uniform as well as permanent; are controlled by an animate entity by reason, that space-time is functionally active, stable and growing. These characteristics combined are indicative of a living entity only, thus, pantheism is a reality.
For time to remain stable and the uniform ‘laws of universal physics’ to be extant could only occur with an animate omnipresent and omnipotent influence.
Those who believe the beauty in nature happened by mere chance are being unrealistic and lack good sense.
Did the complexity of the DNA molecule and the Fibonacci series happen by mere chance or Intelligent Design?
Atheists contribute to the community nothing but emptiness and misdirection while remaining arrogant to the scientific facts and logic. They should try to be open-minded, and face the reality of the universe being organic in nature.
Erwin Schrodinger who mathematically proved that there is no separation, and everything is in the same space and time, it just appears far away. (12 August 1887 – 4 January 1961) Awards: Nobel Prize in Physics, Max Planck Medal.
“Consciousness cannot be accounted for in physical terms. For consciousness is absolutely fundamental. It cannot be accounted for in terms of anything else.”
“What we observe as material bodies and forces are nothing but shapes and variations in the structure of space.” Erwin Schrodinger
Holographic Universe: Cardiff researchers could herald a new era in fundamental physics Media Release Issued: 3rd February 2009 Cardiff University researchers who are part of a British-German team searching the depths of space to study gravitational waves, may have stumbled on one of the most important discoveries in physics according to an American physicist. Craig Hogan, a physicist at Fermilab Centre for Particle Astrophysics in Illinois is convinced that he has found proof in the data of the gravitational wave detector GEO600 of a holographic Universe – and that his ideas could explain mysterious noise in the detector data that has not been explained so far. Feb. 2009
Scientists Prove Existence of God,” German daily Die Welt – Oct 16, 2013
That is where Christoph Benzmüller of Berlin’s Free University and his colleague, Bruno Woltzenlogel Paleo of the Technical University in Vienna, come in. Using an ordinary MacBook computer, they have shown that Gödel’s proof was correct — at least on a mathematical level — by way of higher modal logic.
Having a dull day? Spare a thought for the Universe – it just found out that to outsiders it always looks the one colour … and that colour’s beige! by Karl S. Kruszelnicki.
Scientists Confirm That Reality is an Illusion Our 3D Universe Is a Hologram (the size of a green pea). – YouTube
On Jan. 17, 2000 at 2pm, while crossing a river bridge in Burswood on my bicycle, I observed a velvet textured beige pearl covering the sun. Within the entity there were containers in the shape of elongated, seven-pointed Koch snowflake fractal crystals immersed in a white misty light traveling in a ten o’clock direction. I later deduced that the light flashes on the inner space of the crystals were a binary language conversion pertaining to the senses of all mortals, that is, a holographic universe.
The following day, I imparted this observation to the UN’s High Commissioner for Human Rights, Dr. Mary Robinson and the Hon. Justice Michael Kirby AC CMG of the High Court of Australia.
John Berbatis,
Perth, Australia
Hi,
This is a very important public service announcement concerning our future well being. I found this information very interesting and should be read by everyone. Please take the time to read it over:
We are approaching a future in which they will mandate us to have an RFID microchip implanted in our body. This microchip will contain all our personal information, and we will lose much more of our privacy because of the tracking capabilities.
Did you know this microchiop matches perfectly with prophecy in the Bible? Please read on!
“He causes all, both small and great, rich and poor, free and slave, to receive a mark on their right hand or on their foreheads, and that no one may buy or sell except one who has the mark or the name of the beast, or the number of his name.
Here is wisdom. Let him who has understanding calculate the number of the beast, for it is the number of a man: His number is 666” (Revelation 13:16-18 NKJV).
Referring to the last days, this could only be speaking of a cashless money society, which we have yet to see, but are heading towards. Otherwise we could still buy or sell without the mark among others if physical money was still currency. This mark could not be spiritual, because the word references two different physical locations. If it was spiritual, it would just say in the forehead.
We can see throughout the history of society how we are being conditioned through the process of convenience. More specifically with how we make payments. We went from physical currency to credit and debit cards containing digital currency, to these cards having a microchip in them, to now being able to place your card near a device that will read its data. As well as self check-out lines in our market places and the cryptocurrency industry making a rapid climb in such form as bitcoin. These are all stepping stones for the powers that run this world to bring about their ultimate goal, that is to place a microchip in each and every one of us.
RFID microchip implant technology is on the rise and will be the future of a one world cashless money society.
Continue reading to see how it perfectly matches up with Biblical prophecy!
From a YouTube video titled, “Warning From Man Who Designed RFID Microchip”
Here are some notes from the video:
“Carl Sanders sat in seventeen New World Order meetings with heads-of-state officials such as Henry Kissinger and Bob Gates of the C.I.A. to discuss plans on how to bring about this one-world system. The government commissioned Carl Sanders to design a microchip for identifying and controlling the peoples of the world-a microchip that could be inserted under the skin with a hypodermic needle(a quick, convenient method that would be gradually accepted by society).
Carl Sanders, with a team of engineers behind him, with U.S. grant monies supplied by tax dollars, took on this project and designed a microchip that is powered by a lithium battery, rechargeable through the temperature changes in our skin. Without the knowledge of the Bible(Brother Sanders was not a Christian at the time), these engineers spent one-and-a-half-million dollars doing research on the best and most convenient place to have the microchip inserted.
Guess what? These researchers found that the forehead and the back of the hand(the two places Revelation says the mark will go) are not just the most convenient places, but are also the only viable places for rapid, consistent temperature changes in the skin to recharge the lithium battery. The microchip is approximately seven millimeters in length, .75 millimeters in diameter, about the size of a grain of rice. It is capable of storing pages upon pages of information about you. All your general history, work history, crime record, health history, and financial data can be stored on this chip.
Brother Sanders believes that this microchip, which he regretfully helped design, is the “mark” spoken about in Revelation 13:16-18. The original Greek word for “mark” is “charagma,” which means a “scratch or etching.” It is also interesting to note that the number 666 is actually a word in the original Greek. The word is “chi xi stigma,” with the last part, “stigma,” also meaning “to stick or prick.”
Mr. Sanders asked a doctor what would happen if the lithium contained within the RFID microchip leaked into the body. The doctor replied by saying a terrible sore would appear in that spot. This is what the book of Revelation says: “And the first(Angel) went, and poured out his vial on the earth; and there fell a noisome and grievous sore on the men which had the mark of the beast, and on them which worshipped his image” (Revelation 16:2).
WHAT IS THE NAME OF THE BEAST, THE NUMBER OF ITS NAME? THE MYSTERY OF THE NUMBER 666 REVEALED?
What I first want to mention, before I share my thoughts on the number of the beast, is that God confirms in threes. We can see this throughout scripture: “For there are three that bear witness in heaven: the Father, the Word, and the Holy Spirit; and these three are one” (1 John 5:7 NKJV). “and that He was buried, and that He rose again the third day according to the Scriptures” (1 Corinthians 15:4 NKJV). “…Holy, holy, holy, Lord God Almighty, Who was and is and is to come!” (Revelation 4:8 NKJV). There are many more examples, but I thought I would just share three of them to make the point.
Examining Revelation 13:16-18, the first group of three I would like to point out is that the mark of the beast is described in three separate verses, 16, 17 and 18. The next three I see is in verse 16, “He causes all…” is followed by three contrasting categories of people, “both small and great, rich and poor, free and slave…”. Then unto verse 17, it opens with, “and that no one may buy or sell except one who has…”, followed by three explanations of what one must have to buy or sell, “…the mark or the name of the beast, or the number of his name”. Then in verse 18, we read “Let him who has understanding calculate…”, which is followed by, “the number of the beast, for it is the number of a man: His number is 666”. The last three I see is the number 6 being used three times in a row. The reason I’m making this point is because of the number 666, as you will read below.
“17: even that not any could buy or sell, except the one having the mark, or the name of the beast, or the number of its name” (Literal Greek Translation).
Here we see the mark being identified as having the name of the beast, and that name being identified as being a number. We can know that these attributes being described in verse 17 are all in one thing because the Bible warns of us about receiving the mark, singular. It is clear in verse 17 that we cannot buy or sell without the mark(being previously identified as a microchip), but why cant we buy or sell without the name of the beast, or the number of its name? What does that have to do with us buying or selling if the number of the beast only applies to identifying the Antichrist, as the common thought has been? So we need the number of the beast to buy or sell. What does this mean?
“here the wisdom is, the one having the mind let him calculate the number of the wild beast, number for of human it is, and the number of it 666” (Revelation 13:8 Greek Translation).
“Here is wisdom. Let him that has understanding count the number of the beast: for the number of man; and its number is six hundred sixty-six” (Jubilee Bible 2000 translation).
“In this situation wisdom is needed. Let the person who has insight figure out the number of the beast, because it is a human number. The beast’s number is 666” (GOD’S WORD translation).
The Greek word “anthrōpos” being used in verse 18 where it says “of human” is the Greek strongs concordance G444. The first two definitions of the word are “a human being, whether male or female”, and, “generically, to include all human individuals”. Could the number of the beast apply to all mankind? In the Greek, and other translations, you will notice the beast is described as an “it”, instead of “him”. The reason I’m making this point is because when a translation says “His number is 666”, this would imply a singular person, the Antichrist. But by saying “the number of it 666”, implies that it is of the beast system as a whole.
Throughout the years there have been people trying to calculate numbers based on titles and names that come up to the number 666 to identify one person. But from verse 18, I do not see where God is telling us to count up to 666, but rather to count the number of the beast. This number is identified as 666. So the verse is telling us to count the number 666.
What does it mean to count? It means to add up. So how could we add up 666? Remember my previous point about God confirming in threes. So logically, what would be the best way to count the number 666? To count it equally in threes based off the number. We cannot count it equally as 600+60+6, this would also bring us back to the start. We cannot count it as 600+600+600, or 60+60+60, because there are no zeroes in between or at the end of 666. The only option is 6+6+6=18. What is interesting is that the verse that reveals for us to count the number itself is verse 18, being the third verse out of three verses that describe the mark of the beast. What is 18 divided by 3? 6. So 3×6=18, or 6+6+6=18.
Another interesting point is the only two other combinations(making a total of three possible combinations) for placing a “+” symbol in between the 6’s are 66+6=72, and 6+66=72. Adding 7+2 and 7+2 equals 18. Add both 72’s together and you get 144. Why the number 144 is interesting is because the verse following Revelation 13:18 is the first time in the Bible where the 144,000 are being described in detail: “Then I looked, and behold, a Lamb standing on Mount Zion, and with Him one hundred and forty-four thousand, having His Father’s name written on their foreheads…” (Revelation 14:1).
Now if you add up all three numbers by counting 666 by moving the “+” symbol around, it would be 72+72+18=162. What is interesting about the number 162, is, if you divide 144,000 by 162, you get 888. The name of Jesus in Greek gematria adds up to 888. Revelation 14:1 not only mentions the 144,000, but also the Lamb who is Jesus.
Applying the same format that I used with calculating the number 666, in which I got the numbers 144 and 162, they also bring interesting results bringing us to the number 18:
– 14+4=18*
– 16+2=18*
– 1+4+4=(9), 1+6+2=(9), (9+9)=18*
– 1+44=(45), 1+62=(63), (4+5)=9, (6+3)=9, 9+9=18* | (45+63)=108, 10+8=18*
– 14+4=(18), 1+44=(45), (1+8)=9, (4+5)=9, 9+9=18* | (18+45)=[63] | 16+2=(18), 1+62=(63), (1+8)=9, (6+3)=9, 9+9=18* | (18+63)=[81] | [6+3]=9, [8+1]=9, 9+9=18* | [63+81]=144
– 1+4+4=(9), 14+4=(18), 1+44=(45), (9+18+45)=[72] (any two combinations of (9, 18, 45, and 72 will add to 18*) | 1+6+2=(9), 16+2=(18), 1+62=(63), 9+18+63=[90] (any two combinations of (9, 18, 63, and 90 will get you 18*) | [7+2]=9, [9+0]=9, 9+9=18* | [72+90]=162
Now what is interesting about the number for Jesus, 888, is that if you apply this same formula, you get 8+8+8=24. Why the number 24? Revelation chapter 4 tells us there are 24 elders seated around the throne of God. This is the same throne where Jesus sits.
Now if you take 8+8+8=24, and 8+88=96, and 88+8=96, you get 24+96+96=216. Take 144,000 divided by 216 and you get 666. Remember that this was the same exact formula to get the number 162 out of counting 666 that brought about the number 888 when dividing 144,000 by 162. It is perpetual.
With using the same formula of counting by adding the “+” symbol in between the numbers, why do all these numbers relate in such a way?
Another interesting point to note is that if you add up all the numbers from 1 to 36, it totals 666. The number 36, as in three sixes? Could this be a hint that we should add up three sixes instead of perceiving the number as six-hundred sixty six?
So what could this mean? Well we know in this world we are identified by numbers in various forms. From our birth certificate to social security, as well as our drivers license, being identified based on a system of rulership. So it is possible that this RFID microchip will contain a new identification that has a total of 18 characters. Could this be the name of the beast, the number of its name? The beast system that is identified by 18 characters? This would fit scripture that speaks of a mark that we must have to buy or sell in our right hand or forehead, and that it also contains the number of the beast, during a future cashless money society.
Revelation 13:11-15 tells us that a false prophet will rise up deceiving many to take the mark of the beast because of the miracles he does before men. At all costs do not take it!
“Then a third angel followed them, saying with a loud voice, “If anyone worships the beast and his image, and receives his mark on his forehead or on his hand, he himself shall also drink of the wine of the wrath of God, which is poured out full strength into the cup of His indignation. He shall be tormented with fire and brimstone in the presence of the holy angels and in the presence of the Lamb. And the smoke of their torment ascends forever and ever; and they have no rest day or night, who worship the beast and his image, and whoever receives the mark of his name” (Revelation 14:9-11).
Did you hear about the lady from a major media outlet who reported a major event occurring approximately 20 minutes before it actually happened?
It’s time we know the truth.
A reporter from BBC reported the collapse of World Trade Center 7 on September 11th 2001 approximately 20 minutes before it actually!
Remember, WTC 7 did not get hit by anything, so there would be no reason to assume it would collapse. But she didn’t report on assumption, but that it already did collapse!
What could this mean? The only logical explanation is that the power that orchestrated 9/11 is the same power that controls the major media outlets!
Don’t believe me? Watch the broadcast yourself uploaded on YouTube titled: “BBC Reports 911, WTC 7 Collapse BEFORE it Happens”
It gets even worse…
Did you know there is other footage they don’t want us to see twice? I’m speaking of footage on the day of September 11th 2001 during the time the planes hit both Trade Towers. Eye witness reports say the following concerning the planes:
– “No, it wasn’t a commercial airliner”
– “…but it didn’t have any markings on it that I saw”
– “no emblems, no logos”
– “It definitely did not look like a commercial plane”
– “I did not see any windows on the sides”
– “It was a black plane, it looked like a fighter jet”
– “It was black, it looks like a military plane”
– “It was a military plane”
This video also shares testimonies from firefighters who share their experience being in the world trade towers as bombs were exploding(to weaken the structure of the buildings to cause the free fall collapse).
As a terrorist, how could you smuggle bombs into the trade towers with all of its security systems which includes bomb sniffing dogs? Also, if terrorists did plant the bombs, then why weren’t we told about the bombs?!
You can watch a video of this on YouTube by titled: “9/11 – THE FOOTAGE THEY DIDN’T LET YOU SEE TWICE (9/11 2001 Documentary)”
What about the attack on the Pentagon? Was it really a plane that hit it, or something else, maybe a missle?
Why did the FBI go around and confiscate camera footage from the surrounding buildings not releasing them to the public when they clearly showed us the planes that hit the world trade towers?
Why was the only footage released to the public from one of the most heavily guarded buildings in the world low quality footage showing only five frames at about one frame per second?
The U.S. Secretary of Defense Donald Rumsfeld admitted the day before the attack that the Pentagon was missing 2.3 trillion dollars. Could this attack on the Pentagon have anything to do with covering up that information?
So where am I going with all of this? What’s the purpose of this major event that occurred on September 11th 2001? Why did we go to war with Afghanistan and Iraq?
David Rockefeller is quoted to be saying:
“Some even believe we are part of a secret cabal working against the best interests of the United States, characterizing my family and me as ‘internationalists’ and of conspiring with others around the world to build a more integrated global political and economic structure–one world, if you will. If that’s the charge, I stand guilty, and I am proud of it.” – David Rockefeller, Memoirs
Another video to watch is an infamous interview done with movie producer Aaron Russo titled, “Aaron Russo RFID Human Implant Chip”
Aaron Russo produced such movies as Mad as Hell, Trading Places, and America: Freedom to Fascism. He gained the attention of a man named Nick Rockefeller who saw the movie Made as Hell and knew Aaron was running for governor of Nevada. Knowing the impact Aaron was having on society, who being a freedom fighter standing for the constitution of the United States, Nick sought to befriend him to subtly recruit him into his secret society.
Aaron shares what Nick had told him:
“He’s the one who told me 11 months before 9/11 ever happened that there was going to be an event. He never told me what the event was going to be…and out of that event we were going to invade Afghanistan to run pipelines from the Caspian Sea. We are going to invade Iraq to take over the oil fields to establish a base in the middle east, and make it all part of the New World Order…”
“I remember he was telling me how he was going to see soldiers looking in caves for people in Afghanistan and Pakistan and all these places. And there was going to be this war on terror, where this is no real enemy, and the whole thing is a giant hoax. But it is a way for the government to take over the American people…”
Aaron asked Nick, “What’s the end goal? And he said the end goal is to get everyone chipped to control the whole society. To have the bankers, the elite people controlling the world…”
Visit http://WWW.BIBLEFREEDOM.COM to see all the proof! It is at the point where it takes more faith not to believe that the Bible truly is the word of God!
You will see proof for Noah’s Ark, the Red Sea Crossing, Sodom and Gromorrah, scientific evidence the Bible is inspired by God, testimonies from all walks of life concerning Jesus, and much more!